III.44 Knot Polynomials

W. B. R. Lickorish

1 Knots and Links

A knot is a curve in three-dimensional space that is closed (in other words, it stops where it began) and never meets itself along its way. A link is several such curves, all disjoint from one another, which are called the components of the link. Some simple examples of knots and links are the following:

Two knots are equivalent or “the same” if one can be moved continuously, never breaking the “string,” to become the other. Isotopy is the technical term for such movement. For example, the following knots are the same:

The first problem in knot theory is how to decide whether two knots are the same. Two knots may appear to be very different but how does one prove that they are different? In classical geometry two triangles are the same (or congruent) if one can be moved rigidly on to the other. Numbers that measure side-lengths and angles are assigned to each triangle to help determine whether this is the case. Similarly, mathematical entities called invariants can be associated with knots and links in such a way that if two links have different invariants, then they cannot be the same link. Many invariants relate to the geometry or topology of the complement of a link in three-dimensional space. The FUNDAMENTAL GROUP [IV.6 §2] of this complement is an excellent invariant, but algebraic techniques are then needed to distinguish the groups. The polynomial of J. W. Alexander (published in 1926) is a link invariant derived from distinguishing such groups. Although rooted in ALGEBRAIC TOPOLOGY [IV.6], the Alexander polynomial has long been known to satisfy a skein relation (see below). The HOMFLY polynomial of 1984 generalizes the Alexander polynomial and can be based on the simple combinatorics of skein theory alone.

1.1   The HOMFLY Polynomial

Suppose that links are oriented so that directions, indicated by arrows, are given to all components. To each oriented link L is assigned its HOMFLY polynomial P(L), a polynomial with integer coefficients in two variables υ and z (allowing both positive and negative powers of υ and z). The polynomials are such that

and there is a linear skein relation

This means that equation (2) holds whenever three links have identical diagrams except near one crossing where they are as follows

then this equation holds.

This turns out to be good notation, although one could in principle use x and y in place of υ^-1 and -υ. Although Alexander’s polynomial satisfied a particular instance of (2), it took almost sixty years and the discovery of the Jones polynomial for it to be realized that this general linear relation can be used. Note that there are two possible types of crossing in a diagram of an oriented link. A crossing is positive if, when approaching the crossing along the under-passing arc in the direction of the arrow, the other directed arc is seen to cross over from left to right. If the over-passing arc crosses from right to left, the crossing is negative. When interpreting the skein relation at a crossing of a link L, it is vital that L be regarded as L₊ if the crossing is positive and as L_- if it is negative.

The theorem that underpins this theory, which is not at all obvious, is that it is possible to assign such polynomials to oriented links in a coherent fashion, uniquely, independent of any choice of a link’s diagram. A proof of this is given in Lickorish (1997).

1.2   HOMFLY Calculations

In a diagram of a knot it is always possible to change some of the crossings, from over to under, to achieve a diagram of the unknot. Links can be undone similarly. Using this, the polynomial of any link can be calculated from the above equations, though the length of the calculation is exponential in the number of crossings. The following is a calculation of P(trefoil). Firstly, consider the following instance of the skein relation:

Substituting the polynomial 1 for the polynomials of the two unknots, this shows that the HOMFLY polynomial of the two-component unlink is z^-1(υ^-1 - υ). A second usage of the skein relation is

Substituting the previous answer for the unlink shows that the HOMFLY polynomial of the Hopf link is equal to z^-1(υ^-3 - υ^-1) - zv^-1. Finally, consider the following instance of_/ the skein relation:

Substitution of the polynomial already already calculated for the Hopf link and of course the value 1 for the unknot shows that

P(trefoil) = -υ^-4 + 2υ^-2 + z²υ^-2.

A similar calculation shows that

P(figure eight) = υ² - 1 + υ^-2 - z².

The trefoil and the figure eight thus have different polynomials; this proves they are different knots. Experimentally, if a trefoil is actually made from a necklace (using the clasp to join the ends together) it is indeed found to be impossible to move it to the configuration of a figure eight knot. Note that the polynomial of a knot is not dependent on the choice of its orientation (but this is not so for links).

Reflecting a knot in a mirror is equivalent to changing every crossing in a diagram of the knot from an over-crossing to an under-crossing and vice versa (consider the plane of the diagram to be the mirror). The polynomial of the reflection is always the same as that of the original knot except that every occurrence of υ must be replaced by one of -υ^-1. Thus the trefoil and its reflection,

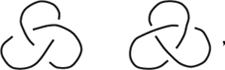
have polynomials

-υ^-4 + 2υ^-2 + z²υ^-2 and -υ⁴ + 2υ² + z²υ².

As these polynomials are not the same, the trefoil and its reflection are different knots.

2   Other Polynomial Invariants

The HOMFLY polynomial was inspired by the discovery in 1984 of the polynomial of V. F. R. Jones. For an oriented link L, the Jones polynomial V(L) has just one variable t (together with t^-1). It is obtained from P(L) by substituting υ = t and z = t^1/2 - t^-1/2, where t^1/2 is just a formal square root of t. The Alexander polynomial is obtained by the substitution υ = 1, z = t^-1/2 - t^1/2. This latter polynomial is well understood in terms of topology, by way of the fundamental group, covering spaces, and homology theory, and can be calculated by various methods involving determinants. It was J. H. Conway who, in discussing in 1969 his normalized version of the Alexander polynomial (the polynomial in one variable z obtained by substituting υ = 1 into the HOMFLY polynomial), first developed the theory of skein relations.

There is one more polynomial (due to L. H. Kauffman) based on a linear skein relation. The relation involves four links with unoriented diagrams differing as follows:

There are examples of pairs of knots that the Kauffman polynomial but not the HOMFLY polynomial can distinguish and vice versa; some pairs are not distinguished by any of these polynomials.

2.1   Application to Alternating Knots

For the Jones polynomial there is a particularly simple formulation, by means of “Kauffman’s bracket polynomial,” that leads to an easy proof that the Jones (but not the HOMFLY) polynomial is coherently defined. This approach has been used to give the first rigorous confirmation of P. G. Tait’s (1898) highly believable proposal that a reduced alternating diagram of a knot has the minimal number of crossings for any diagram of that knot. Here “alternating” means that in going along the knot the crossings go: … over, under, over, under, over, …. Not every knot has such a diagram. “Reduced” means that there are, adjacent to each crossing, four distinct regions of the diagram’s planar complement. Thus, for example, any nontrivial reduced alternating diagram is not a diagram of the unknot. Also, the figure eight knot certainly has no diagram with only three crossings.

2.2   Physics

Unlike that of Alexander, the HOMFLY polynomial has no known interpretation in terms of classical algebraic topology. It can, however, be reformulated as a collection of state sums, summing over certain labelings of a knot diagram. This recalls ideas from statistical mechanics; an elementary account is given in Kauffman (1991). An amplification of the whole HOMFLY polynomial theory leads into a version of conformal field theory called topological quantum field theory.

Further Reading

Kauffman, L. H. 1991. Knots and Physics. Singapore: World Scientific.

Lickorish, W. B. R. 1997. An Introduction to Knot Theory. Graduate Texts in Mathematics, volume 175. New York: Springer.

Tait, P. G. 1898. On knots. In Scientific Papers, volume I, pp. 273-347. Cambridge: Cambridge University Press.