
Chapter Twenty-Two — Conic Sections
The Humongous Book of Algebra Problems
506
Hyperbolas
Transverse and conjugate axes, foci, vertices, and asymptotes
Note: Problems 22.33–22.36 refer to the hyperbola graphed in Figure 22-7 below.
Figure 22-7: The graph of a hyperbola with vertices (–4,3) and (4,3).
22.33 Identify the center of the hyperbola.
The center, (h,k), of a hyperbola is the midpoint of the segment connecting the
vertices. Substitute x
1
= –4, y
1
= 3, x
2
= 4, and y
2
= 3 into the midpoint formula.
The center of the hyperbola is (0,3).
Note: Problems 22.33–22.36 refer to Figure 22-7, the hyperbola graphed in Problem 22.33.
22.34 Calculate the lengths of the transverse and conjugate axes.
The transverse axis of a hyperbola is the segment whose endpoints are the
vertices. According to Figure 22-7, the vertices of the hyperbola are (–4,3) and
(4,3). The length, l
t
, of the horizontal transverse axis is equal to the absolute
value of the difference of the x-values.
If you know
the endpoints
of a VERTICAL
segment, you can
nd its length by
subtracting the y-
values and taking
the absolute
value.

Chapter Twenty-Two — Conic Sections
The Humongous Book of Algebra Problems
507
Therefore, the transverse axis is 8 units long. To calculate the length of the
conjugate axis—a segment perpendicular to the transverse axis at the center
of the hyperbola—draw vertical segments that extend from the vertices to
the asymptotes of the graph. Plot the intersection points of those two vertical
segments and the asymptotes. Connect the four intersection points to form a
rectangle, as illustrated by Figure 22-8.
Figure 22-8: The transverse axis bisects the rectangle horizontally, with endpoints at
the vertices. The conjugate axis bisects the hyperbola vertically, with endpoints (0,2) and
(0,4).
The length, l
c
, of the vertical conjugate axis is the absolute value of the
difference of the endpoints’ y-values.
Note: Problems 22.33–22.36 refer to Figure 22-7, the hyperbola graphed in Problem 22.33.
22.35 Write the equation of the hyperbola in standard form.
The standard form of a hyperbola with a horizontal transverse axis is
, such that the center is (h,k), the distance between the
center and a vertex is a, and the distance between the center and an endpoint of
the conjugate axis is b.
According to Problem 22.33, the transverse axis is 8 units long, so a = 8 ÷ 2 = 4.
The conjugate axis is 2 units long, so b = 2 ÷ 2 = 1. According to Problem 22.33,
the center of the hyperbola is (0,3), so h = 0 and k = 3. Substitute a = 4, b = 1,
h = 0, and k = 3 into the standard form equation.
The transverse
axis is horizontal, so
draw vertical lines
up and down from
both vertices that
stop at the dotted
asymptotes. Those
points are the corners
of the rectangle in
Figure 22-8.
The center
is the midpoint of
both the transverse
and conjugate axes.
Dividing the length of the
transverse axis in half
gives you a, and dividing
the length of the
conjugate axis in half
gives you b.
Chapter Twenty-Two — Conic Sections
The Humongous Book of Algebra Problems
508
Note: Problems 22.33–22.36 refer to Figure 22-7, the hyperbola graphed in Problem 22.33.
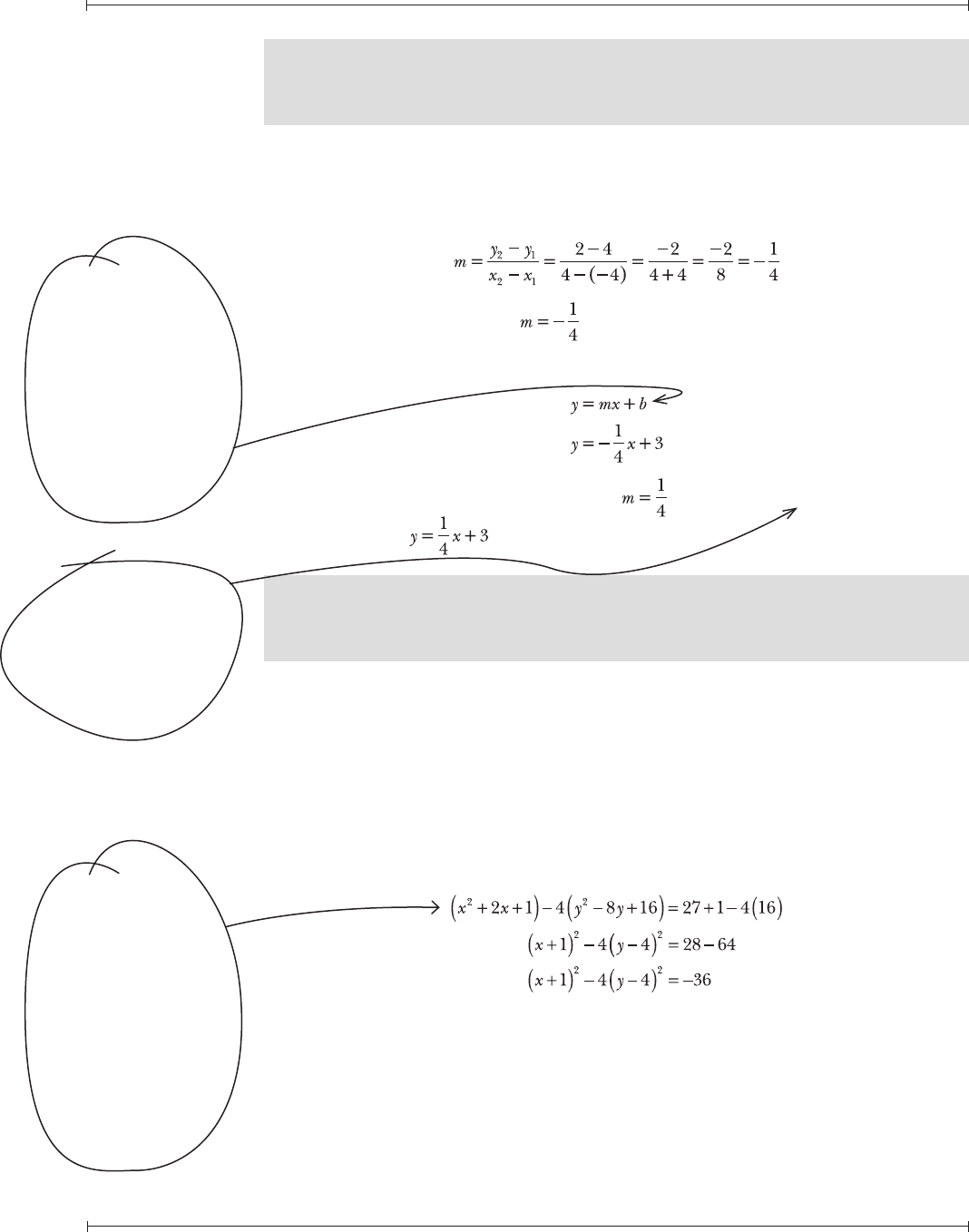
22.36 Write the equations of the asymptotes in slope-intercept form.
Consider the rectangle in Figure 22-8; its corners are also points on the linear
asymptotes. For instance, the asymptote passing through the upper-left and
lower-right corners of the rectangle passes through points (–4,4) and (4,2).
Apply the slope formula to calculate the slope of that asymptote.
The slope of the line is . The asymptote intersects the y-axis at the
center of the hyperbola, (0,3); therefore, its y-intercept is 3. Apply the slope-
intercept form of a line.
The remaining asymptote has slope and y-intercept 3. Thus, it has
equation .
Note: Problems 22.37–22.40 refer to the hyperbola with equation x
2
– 4y
2
+ 2x + 32y – 27 = 0.
22.37 Write the equation in standard form.
Group the x-terms in a set of parentheses, group the y-terms in a second set of
parentheses, and move the constant to the right side of the equation.
(x
2
+ 2x) + (–4y
2
+ 32y) = 27
The coefficients of both squared terms must equal 1, so factor the coefficient of
y
2
out of the corresponding quantity.
(x
2
+ 2x) – 4(y
2
– 8y) = 27
Complete the square for each parenthetical quantity.
The right side of the equation of a hyperbola in standard form must equal 1, so
divide both sides of the equation by –36.
This b is the
y-intercept value
(b = 3), NOT the
b used to represent
the distance
between the center
of the hyperbola and
an endpoint of the
conjugate axis.
Asymptotes
don’t always
have the same y-
intercept. These happen
to because they
intersect on the
y-axis.
Add 1 to the
x-expression
and 16 to the y-
expression. However,
the y-expression is
multiplied by –4,
so you’re actually
adding –4(16) = –64
to both sides of
the equation.

Chapter Twenty-Two — Conic Sections
The Humongous Book of Algebra Problems
509
The positive rational expression must precede the negative rational expression
in the standard form of a hyperbola.
Note: Problems 22.37–22.40 refer to the hyperbola with equation x
2
– 4y
2
+ 2x + 32y – 27 = 0.
22.38 Identify the center and vertices and sketch the graph of the hyperbola.
According to Problem 22.37, the standard form of the equation is
. Because the fraction containing the y-expression is
positive, the transverse axis of this hyperbola is vertical.
The standard form of a hyperbola with a vertical transverse axis is
. Therefore, the center of the hyperbola is (h,k) = (–1,4),
a = 3, and b = 6. Note that a is not necessarily greater than b, unlike in the
equations of ellipses.
Plot the points three units above and below the center and the points six units
left and right of the center. Draw a rectangle whose sides pass through those
four points, as illustrated by Figure 22-9.
The hyperbola
opens up and down.
When the x-expression
is positive (like in
Problems 22.33–22.36)
the transverse axis
is horizontal and the
hyperbola opens left
and right.
a is
the square
root of the
positive fraction’s
denominator
and b
is the square root of
the negative fraction’s
denominator
. It
doesn’t matter
which is
larger.
a
2
is below
the y-expression, so you go a = 3
units up and down ( because y’s measure
vertical distance). Similarly, b
2
is below the
x-expression, so you go b = 6 units left and
right (because x’s measure horizontal
distance).
Put the
positive fraction
in front of the
negative
fraction.

Chapter Twenty-Two — Conic Sections
The Humongous Book of Algebra Problems
510
Figure 22-9: The segment that bisects this rectangle vertically is the transverse axis of
the hyperbola. The segment that bisects the rectangle horizontally is the conjugate axis.
Draw the asymptotes of the hyperbola, the pair of lines that extends through
opposite corners of the rectangle in Figure 22-9. The points a = 3 units
above and below the center, along the transverse axis, are the vertices of the
hyperbola: (–1,1) and (–1,7). The graph passes through each vertex, bends away
from the center point, and approaches (but does not intersect) the asymptotes,
as illustrated in Figure 22-10.
Figure 22-10: The graph of x
2
– 4y
2
+ 2x + 32y – 27 = 0 has a vertical transverse axis
and vertices (–1,1) and (–1,7). Note that the rectangle used to construct the graph is not
part of the graph.
If you
need a more
exact graph, solve
the equation for x
and make a table of
values that includes y-
values greater than 7
and less than 1 (the
y-values of the
vertices).
..................Content has been hidden....................
You can't read the all page of ebook, please click here login for view all page.
