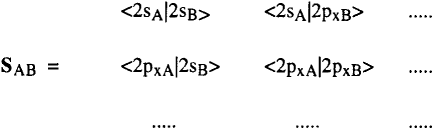1.1 The first period

The algebraic manipulation of atomic orbitals to which the name of hybridization has been attached was initiated by Pauling [2]. Merging together the idea of shared electron pairs of the classical valence theory of Lewis and the quantum mechanical treatment newly presented by Heitler and London for the hydrogen molecule [3], he offered a somewhat intuitive description of the chemical bond in complex molecules by using “hybrid orbitals of maximum bond-forming power” [4]. More concretely, the four valences of the carbon atom in the methane molecule were assigned to four sp³ orbitals whose axes of maximum electron density were arranged in conformity with the dictum [5] “There is a large amount of experimental evidence suggesting that the carbon atom has four valencies radiating from the centre to the corners of a regular tetrahedron”.

In subsequent independent papers, Pauling [4] and Slater [6] generalized the valence-bond treatment made for the H₂ molecule to polyatomic systems as H₂O, NH₃, CH₄ etc … where an atom of the first period (the second row) is linked to hydrogens by several two-electron bonds ; they described the valence orbitals coming from the central atom by appropriate s and p combinations known later as hybrid orbitals. At the same time Hund [7] and Mulliken [8] presented another quantum theory of valence, the molecular orbital method in LCAO form, using the spectroscopic concept of molecular configuration built from s, p, d …pure atomic orbitals. The actual status of the hybridization process was clarified by Van Vleck [9], who showed that the various approximations introduced in the quantum mechanical treatment of valence were responsible for many differences of interpretation rather than the content of the methods themselves. For instance, hybridization involved in the perfect pairing of localized electron-pairs and resonance limited to chemically significant bond functions amount the same thing [10,11] This culminates in the final equivalence of methods starting from common sets of -pure or mixed- atomic orbitals (i.e., valence bond or molecular orbital treatments including all the possible distributions of electrons in the wave function) first proved for very simple systems [12,13] and later generalized [14,15].

1.2 Valence state theory

From the point of view of Natural Philosophy, the concept of atomic valence states, developed by Van Vleck, Mulliken and others [9,16,17,18] in close connection with hybridization, is a major achievement, for it gives a microscopic picture of the atom in situ taking into account the level of complexity to be preserved in the theoretical analysis of chemical facts [1]. The basic ingredient of the theory consists in a formal decoupling of some paired electrons for the atom considered in accordance with its valence in molecules : To do that, two steps may be necessary : first, promoting one electron of a 2s filled subshell, if necessary, into the adjacent p shell (case of carbon s²p² versus s¹p¹p¹) or d shell (case of transition metals s²dⁿ⁻² versus s¹dⁿ⁻¹) ; second, randomizing the spins of the valence electrons with respect to each other (i.e., by means of the value 1/2 K_ij assigned to the exchange term corresponding to the valence orbitals of the atoms in question [16]).

The valence state definition above is just operative, for it generates energy terms calculable from spectroscopic data in the frame of the Slater model of atoms in their neutral and adjacent ionized states. The valence orbitals can be defined in pure or hybrid forms, corresponding to familiar s,p tetrahedral (te), trigonal (tr) and digonal (di) sets for light atoms, or to the huge variety of s,p,d combinations for heavier atoms [19,20]. To conclude by a fairly common remark on the valence states, we will add the fact that their building-up process from atomic experimental data, the F_k and G_k Slater parameters [21], implies that they do not be thought as physical observables, but rather to a linear combination of genuine spectroscopic states [22]. Without going into details of chemistry, the energy loss due to the contribution of atomic excited states in this mixture is said to be largely paid by the formation of bonds.

1.3 The present status of hybridization

Nowadays, the concept of hybridization remains the basis of the most popular description of the molecular bonding, namely that of σ, π - and δ -chemical bonds between atoms in organic [23] as well as inorganic compounds and solids [24]. The terminology of hybridization gives us a simple way to characterize “atoms in molecules” [25] by their valence states, which in the same time precises the type of geometry of the atomic site considered. We can use hybridization not only to sketch the spatial distribution of the binding electron pairs, but also according to Pauling to describe lone pairs with privileged directions depending on their percentage of s and p characters [26,27]. If, finally, we pass over the questions connected to its theoretical origin, hybridization can be considered as a simple way for describing, both in speaking and in writing, the so-called molecular observables in terms of atomic components.

Another reason for the success of such interpretations lies in the fact that hybridization becomes a flexible model applicable to systems of arbitrary shape when it is equipped with the maximum overlapping principle establishing a link between bond strengths and overlap integrals of hybrid orbitals centered on neighbouring atoms [28,29]. As it will be shown in the next section, this is the guiding idea of Murrell [30], Coulson [31] and Del Re [32] for a priori calculations of hybrids in a molecule. In the seventies, however, hybridization has been fiercely criticized by people pretexting that its use supposes a preliminary knowledge of the molecular structure to be predicted and preferring the VSEPR orbital-free model of Gillespie instead [33]. In so far as a qualitative picture of the molecular structure is requested, it would be better to say that both models are basically isomorphous, mimicking molecules by a set of initially equivalent sites whose interaction is afterwards governed by an energy criterion (i.e., the maximum overlapping principle of hybridization, or the hierarchy of electron-pair repulsions in the VSEPR model [34]). By no means, the quantum-chemical applications of the hybridization concept are really concerned in this quarrel ignoring the fact it is first of all a procedure intended to prepare atoms in a molecule to bonding. To end the matter, suffice it to speak in measured terms of hybridization : i) it is a way for constructing “chemical orbitals” , that is to say wave functions which preserve the concept of bond properties [35,36] and in the same time gives us good starting points for the developpement of various semi-empirical or ab initio treatments-, ii) it is not an experimentally observable phenomenon, but according to Coulson merely “a feature of a theoretical description”.

To avoid possible problems of linear dependence, it is generally assumed that the hybrid orbitals h_Ai centered on a given atomic site A are orthonormal:

$<{{\mathrm{h}}_{\mathrm{A}}}_{\mathrm{i}}|{{\mathrm{h}}_{\mathrm{A}}}_{\mathrm{j}}>={\mathrm{\delta }}_{\mathrm{ij}}$

If so, the direction and form of hybrids starting from the central atom A of a highly symmetrical system, as methane or complexes of transition metals, for instance [Co(NH₃)₆]^3 −, are defined by the geometry of the molecule, independently of the nature of the nearest neighbours B. In such cases, hybridization of orbitals on atom A gives us a convenient basis for describing the set of equivalent bonds A-B by combination with the orbitals of atoms B ; hybrids of A adapted to the symmetry of the molecule (i.e., to its directed valences) are easily determined by projecting a set of central-field orbitals s,p,d … of atom A into the irreducible representations of the point group to which the molecule belongs [19]. Lists of all the possible types of s,p,d hybrids for highly symmetrical molecules and a number of s, p, d, f combinations are given in the literature [37,38]. Hybrids for some compounds of lower symmetry have been also reported [39,40].

More generally, hybrids pointing in any direction around an atomic site A can be obtained by straightforward geometrical constructions [41] provided the form of the molecule is already known (i.e., by another theoretical or experimental way). The vectorial character of the p_x, p_y, p_z orbitals transforming as the axes of a cartesian coordinate system ensures the success of such calculations, except if the valence angles become smaller than 90° : A well known example is cyclopropane, a three-membered ring with bond angles of 60° to be described according to Coulson and Moffitt [42] by a model of hybridized “banana bonds” explaining its conjugation properties as a substituent on aromatic nuclei. A somewhat similar situation occurs with four-membered rings, as cyclobutane [43]. It has been suggested [44] that the difficulties leading to bent banana bonds in small rings could be circumvented by accepting combinations with complex coefficients as solutions for hybrids. The difficulties due to the occurrence of broken symmetry solutions with respect to time reversibility when using complex wave functions can be solved by separating real and imaginary parts in variational caculations [45]. However, bent bonds formed from real orbitals are a little more convenient [46].

If we limit ourselves to s and p combinations, we can state without any doubt that the bonds formed between an atom A and its neighbours B using appropriate geometrical hybrids verify the principle of “maximum overlapping of atomic orbitals” (M.O.A.O. criterion) even if the atoms B linked to A are different. Clearly, the integrated product h_Ah_B of a pair of σ hybrid orbitals giving the best bond energy E_AB will be as large as possible if the directions of h_A and Jig in space coincide, the overlap between h_A and h_B being maximum. However, hybridization of d (and f ) put some problems to theoreticians, for instance the preservation of the cylindrical symmetry properties of s p d hybrids [47] and the unicity of possible solutions [48], but they are not serious according to Pauling [49]. The alternative suggested by Daudel and Bucher [50] was relaxing the orthogonality constraints.

2.2 Maximum overlap criteria : The Del Re method

Methods for a priori determination of atomic hybrids using the principle of maximum overlapping have been implemented towards the sixties, either in global form or in a more local form. The procedures used by Murrell for quasi centro-symmetrical molecules [30] and by Coulson and Goodwin [31] consist in simply maximizing the sum of all overlap integrals between σ paired orbitals. More or less different techniques have been suggested later [51,52] The method developed by Del Re from 1963 [32] is a more refined multi-local treatment involving each atom and its next neighbours in turn. Taking the likeness of the eigenvectors of the overlap and effective Hamiltonian matrices for granted as a consequence of the possibility of evaluating off-diagonal terms approximately by means of the Mulliken proportionality rule to overlap (see [53,54]), this procedure is based on a principle of “maximum localization of hybrid orbitals” (M.L.H.O. criterion). The best hybrids are the ones which lead to a localization of the overlap matrix of the whole molecule, under the condition that the set of orbitals assigned in this manner to each atom be orthonormal. Here, the word “localization” means that the resulting Hamiltonian is -to a certain degree of approximation- factorized in 2×2 blocks corresponding to the various pairs of bonded atoms, except if the presence of several large off-diagonal elements suggests the idea of many-center bonds. Such a definition gives us a unique set of hybridized functions for each atom of a molecule consistent with the maximum overlapping criterion and in the same time an efficient computational procedure for any system. The equations of the Del Re hybridization method have been written out first in the case of single-zeta s, p valence orbitals of first-row atoms and generalized later to more complicated basis sets, as it will be discussed below.

2.2.1 Building procedure of maximum localization hybrid orbitals

Consider a system composed of atoms A, B, C etc … and let χ_A, χ_B, χ_C be their respective 2s, 2p_x, 2p_y , 2p_z valence orbitals defined in a single coordinate system. The basis set of the whole molecule can be represented by the row vector χ = (χ_A , χ_B , χ_C etc …) collecting all these basis functions :

$\mathrm{\chi }=\left(2{\mathrm{s}}_{\mathrm{A}}{{2\mathrm{p}}_{\mathrm{x}}}_{\mathrm{A}}2{\mathrm{p}}_{\mathrm{yA}}2{\mathrm{p}}_{\mathrm{zA}}2{\mathrm{s}}_{\mathrm{B}}{{2\mathrm{p}}_{\mathrm{x}}}_{\mathrm{B}}{{2\mathrm{p}}_{\mathrm{y}}}_{\mathrm{B}}{{2\mathrm{p}}_{\mathrm{z}}}_{\mathrm{B}}\dots \right)$

  (2)

The overlap matrix S = (χ^†, χ) formed of diagonal elements AA, BB all equal to the 4×4 unit matrix I and of off-diagonal blocks AB, AC etc … such as S_AB = (χ^†A, χ_Β) can be written as follows :

$\mathbf{S}=\begin{array}{cccc}\hfill \mathbf{I}\hfill & \hfill {\mathbf{S}}_{\mathrm{AB}}\hfill & \hfill {\mathbf{S}}_{\mathrm{AC}}\hfill & \hfill \dots \hfill \\ \hfill \dots \hfill & \hfill \mathbf{I}\hfill & \hfill {\mathbf{S}}_{\mathrm{BC}}\hfill & \hfill \dots \hfill \\ \hfill \dots \hfill & \hfill \dots \hfill & \hfill \mathbf{I}\hfill & \hfill \dots \hfill \end{array}$

  (3)

where S_AB, S_ACetc … are the overlap matrices between pure atomic orbitals of A , B , C etc …

${\mathbf{S}}_{\mathrm{AB}}=\begin{array}{ccc}\hfill <2{\mathrm{s}}_{\mathrm{A}}|2{\mathrm{s}}_{\mathrm{B}}>\hfill & \hfill <2{\mathrm{s}}_{\mathrm{A}}|2{{\mathrm{p}}_{\mathrm{x}}}_{\mathrm{B}}>\hfill & \hfill .....\hfill \\ \hfill <2{{\mathrm{p}}_{\mathrm{x}}}_{\mathrm{A}}|2{\mathrm{s}}_{\mathrm{B}}>\hfill & \hfill <2{{\mathrm{p}}_{\mathrm{x}}}_{\mathrm{A}}|2{{\mathrm{p}}_{\mathrm{x}}}_{\mathrm{B}}>\hfill & \hfill .....\hfill \\ \hfill .....\hfill & \hfill .....\hfill & \hfill .....\hfill \end{array}$

  (4)

We have to find a unitary transformation U changing the χ basis into a new one χ’ such as U only mixes the orbitals belonging to a same atom without mixing them with those of other atoms. Then the χ’ basis is changed into

$\mathrm{\chi }=\left({\mathrm{\chi }}_{\mathrm{A}}{\mathbf{U}}_{\mathrm{A}}{\mathrm{\chi }}_{\mathrm{B}}{\mathbf{U}}_{\mathrm{B}}{\mathrm{\chi }}_{\mathrm{C}}{\mathbf{U}}_{\mathrm{C}}\mathit{etc}\dots \right)$

  (5)

and S_AB and S into

${\mathbf{S}}_{\mathrm{AB}}^{\mathbf{\prime }}={{\mathbf{U}}^{†}}_{\mathrm{A}}{\mathbf{S}}_{\mathrm{AB}}{\mathbf{U}}_{\mathrm{B}}$

  (6)

${\mathbf{S}}^{\mathbf{\prime }}={\mathbf{U}}^{†}\mathbf{SU}=\begin{array}{cccc}\hfill \mathbf{I}\hfill & \hfill {{\mathbf{U}}^{\mathbf{†}}}_{\mathrm{A}}{\mathbf{S}}_{\mathrm{AB}}{\mathbf{U}}_{\mathrm{B}}\hfill & \hfill {{\mathbf{U}}^{\mathbf{†}}}_{\mathrm{A}}{\mathbf{S}}_{\mathrm{AC}}{\mathbf{U}}_{\mathrm{C}}\hfill & \hfill \dots \hfill \\ \hfill \dots \hfill & \hfill \mathbf{I}\hfill & \hfill {{\mathbf{U}}^{\mathbf{†}}}_{\mathrm{B}}{\mathbf{S}}_{\mathrm{BC}}{\mathbf{U}}_{\mathrm{C}}\hfill & \hfill \dots \hfill \\ \hfill \dots \hfill & \hfill \dots \hfill & \hfill \mathbf{I}\hfill & \hfill \dots \hfill \end{array}$

  (7)

The localization would be perfect if each of the off-diagonal blocks S′_AB, S′_AC… contains only one non-zero element on its diagonal, say λ_AB λ_AC… , for a given hybrid of atom A should form a bond with only one hybrid belonging to each of its neighbouring atoms B, C … Therefore, the U transformation has to keep the diagonal blocks of S unchanged and to transform the remaining part of the S matrix in order to have only one element in each row and column. It is always possible to arrange the hybrid orbitals of any (polyatomic) molecule in such a way that the overlaps < h_AB | h_BA > , < h_AC | h_CA > etc … are on the diagonal of their S′_AB S′_AC etc… respective blocks. The maximum overlap condition will be practically satisfied if all the S′_xy blocks are as close as possible to diagonal matrices, that is to say if the S′ whole matrix has the following form:

${\mathbf{S}}^{\mathbf{\prime }}=\begin{array}{ccccccccc}\hfill 1\hfill & \hfill 0\hfill & \hfill 0\hfill & \hfill 0\hfill & \hfill {\mathrm{\lambda }}_{\mathrm{AB}}\hfill & \hfill \dots \hfill & \hfill \dots \hfill & \hfill \dots \hfill & \hfill \dots \hfill \\ \hfill 0\hfill & \hfill 1\hfill & \hfill 0\hfill & \hfill 0\hfill & \hfill \dots \hfill & \hfill \dots \hfill & \hfill \dots \hfill & \hfill \dots \hfill & \hfill \dots \hfill \\ \hfill 0\hfill & \hfill 0\hfill & \hfill 1\hfill & \hfill 0\hfill & \hfill \dots \hfill & \hfill \dots \hfill & \hfill \dots \hfill & \hfill \dots \hfill & \hfill \dots \hfill \\ \hfill 0\hfill & \hfill 0\hfill & \hfill 0\hfill & \hfill 1\hfill & \hfill \dots \hfill & \hfill \dots \hfill & \hfill \dots \hfill & \hfill \dots \hfill & \hfill \dots \hfill \\ \hfill {\mathrm{\lambda }}_{\mathrm{BA}}\hfill & \hfill \dots \hfill & \hfill \dots \hfill & \hfill \dots \hfill & \hfill 1\hfill & \hfill 0\hfill & \hfill 0\hfill & \hfill 0\hfill & \hfill \dots \hfill \\ \hfill \dots \hfill & \hfill \dots \hfill & \hfill \dots \hfill & \hfill \dots \hfill & \hfill 0\hfill & \hfill 1\hfill & \hfill 0\hfill & \hfill 0\hfill & \hfill \dots \hfill \\ \hfill \dots \hfill & \hfill \dots \hfill & \hfill \dots \hfill & \hfill \dots \hfill & \hfill 0\hfill & \hfill 0\hfill & \hfill 1\hfill & \hfill 0\hfill & \hfill \dots \hfill \\ \hfill \dots \hfill & \hfill \dots \hfill & \hfill \dots \hfill & \hfill \dots \hfill & \hfill 0\hfill & \hfill 0\hfill & \hfill 0\hfill & \hfill 1\hfill & \hfill \dots \hfill \\ \hfill \dots \hfill & \hfill \dots \hfill & \hfill \dots \hfill & \hfill \dots \hfill & \hfill \dots \hfill & \hfill \dots \hfill & \hfill \dots \hfill & \hfill \dots \hfill & \hfill \dots \hfill \end{array}$

  (8)

where the terms of the off-diagonal blocks explicitely written are those having the largest absolute values for bond-hybrid overlaps. For instance, we have:

${\left({{\mathbf{U}}^{†}}_{\mathrm{A}}{{\mathbf{S}}_{\mathrm{A}}}_{\mathrm{B}}{\mathbf{U}}_{\mathrm{B}}\right)}_{11}={\mathrm{\lambda }}_{\mathrm{AB}}\mathrm{for}\mathrm{the}\mathrm{AB}\mathrm{block}$

${\left({{\mathbf{U}}^{†}}_{\mathrm{A}}{{\mathbf{S}}_{\mathrm{A}}}_{\mathrm{B}}{\mathbf{U}}_{\mathrm{B}}\right)}_{11}={\mathrm{\lambda }}_{\mathrm{BA}}\mathrm{for}\mathrm{the}\mathrm{BA}\mathrm{block}$

  (9)

with λ_BA = λ_AB. Putting U^†_A1 and U^†_Bl for the first rows of U^†_A and U^†_B , that is to say :

${{\mathbf{U}}^{†}}_{\mathrm{A}1}{{\mathbf{S}}_{\mathrm{A}}}_{\mathrm{B}}{\mathbf{U}}_{\mathrm{B}}=\left({\mathrm{\lambda }}_{\mathrm{AB}}\right)\times \left(1,0,0,0\right)$

  (10)

${{\mathbf{U}}^{†}}_{\mathrm{B}1}{\mathbf{S}}^{†}{{}_{\mathrm{A}}}_{\mathrm{B}}{\mathbf{U}}_{\mathrm{A}}=\left({\mathrm{\lambda }}_{\mathrm{AB}}\right)\times \left(1,0,0,0\right)$

  (11)

and multiplying Eqs (10) and (11) on the right by U^†_B and U^†_A respectively, we get

${{\mathbf{U}}^{†}}_{\mathrm{A}1}{{\mathbf{S}}_{\mathrm{A}}}_{\mathrm{B}}={\mathrm{\lambda }}_{\mathrm{AB}}{{\mathbf{U}}^{†}}_{\mathrm{B}1}$

  (12a)

${{\mathbf{U}}^{†}}_{\mathrm{B}1}{\mathbf{S}}^{†}{{}_{\mathrm{A}}}_{\mathrm{B}}={\mathrm{\lambda }}_{\mathrm{AB}}{{\mathbf{U}}^{†}}_{\mathrm{A}1}$

  (12b)

or after multiplying Eq. (12a) by S^†_AB, substituting Eq. (12b) and transposing :

$\begin{array}{l}{\mathbf{S}}_{\mathrm{AB}}{{\mathbf{S}}^{†}}_{\mathrm{AB}}{\mathbf{U}}_{\mathrm{A}1}={{\mathrm{\lambda }}^{{}_2}}_{\mathrm{AB}}{\mathbf{U}}_{\mathrm{A}1}\end{array}$

  (13)

Similarly,

$\begin{array}{l}{{\mathbf{S}}^{†}}_{\mathrm{AB}}{\mathbf{S}}_{\mathrm{AB}}{\mathbf{U}}_{\mathrm{B}1}={{\mathrm{\lambda }}^{{}_2}}_{\mathrm{AB}}{\mathbf{U}}_{\mathrm{B}1}\end{array}$

Thus, U_A1 and U_B1 are the eigenvectors associated with the largest eigenvalues of S_ABS^†_AB and S^†_ABS_AB respectively. If we now pass to the pair of atoms A C, we can apply the same treatment to the S′_AC block matrices, so that we have a new pair of equations defining U_A2 and U_C1 and so on … This process has be repeated for each atom, giving a number of conditions depending on its number of valence orbitals and next-neighbours (here, four).

The eigenvectors computed from the largest eigenvalues of Eq. (13), say Û_A1, Û_A2, Û_A3, Û_A4, Û_B1, Û_B2, Û_B3, Û_B4 etc …, give only a first approximation Û of the unitary transformation U we wanted. It is due to the fact that the local transformations Û_A, Û_B defined in this way for the various atoms A, B etc…are not unitary because the overlap matrices connecting each of them with its neighbours do not generally commute. This deficiency can be cured by substituting unitary transformations U_A, U_B for Û_A, Û_B etc … as close as possible from the latter (i.e., by requiring that the scalar product of the Û_A and U_A vectors is maximum) Using Eq. (13), the corresponding variational problem writes:

$\mathrm{\delta }\left\{{\displaystyle {\sum }_{\mathrm{i}}\left[{{\mathrm{\lambda }}^2}_{\mathrm{Ai}}\left({{\widehat{\mathbf{U}}}^{†}}_{\mathrm{Ai}}{\mathbf{U}}_{\mathrm{Ai}}+{{\mathbf{U}}^{†}}_{\mathrm{Ai}}{\widehat{\mathbf{U}}}_{\mathrm{Ai}}\right)-{\displaystyle {\sum }_{\mathrm{j}}·}{\mathbf{M}}_{\mathrm{ij}}{{\mathbf{U}}^{†}}_{\mathrm{Ai}}{\mathbf{U}}_{\mathrm{Aj}}\right]}\right\}=0$

  (14)

with a symmetrical set M_A of Lagrange multipliers M_ij preserving the unitary character of the U_A transformations. Differentiating Eq. (14), we get a couple of transposed equations such as:

${\widehat{\mathbf{U}}}_{\mathrm{A}}{{\Lambda }^2}_{\mathrm{A}}={\mathbf{M}}_{\mathrm{A}}{\mathbf{U}}_{\mathrm{A}}$

  (15)

where ${{\Lambda }^2}_{\mathrm{A}}$ is a diagonal matrix of elements

${{\mathrm{\lambda }}^2}_{\mathrm{A}1}={{\mathrm{\lambda }}^2}_{\mathrm{AB}},{{\mathrm{\lambda }}^2}_{\mathrm{A}2}={{\mathrm{\lambda }}^2}_{\mathrm{AC}},\mathit{etc}\dots$

  (16)

We can solve them by assuming that the U_A blocks forming the U unknown transformation are the products of two new unitary matrices V_A and W^†_A , the first one permitting by the same token to reduce M_A to its diagonal form m_A:

${\mathbf{U}}_{\mathrm{A}}={\mathbf{V}}_{\mathrm{A}}{{\mathbf{W}}^{†}}_{\mathrm{A}}$

  (17a)

${\mathbf{M}}_{\mathrm{A}}={\mathbf{V}}_{\mathrm{A}}{\mathbf{m}}_{\mathrm{A}}{{\mathbf{V}}^{†}}_{\mathrm{A}}$

  (17b)

Then, Eq. (15) and its transpose are replaced by

${\widehat{\mathbf{U}}}_{\mathrm{A}}{{\Lambda }^2}_{\mathrm{A}}={\mathbf{V}}_{\mathrm{A}}{\mathbf{m}}_{\mathrm{A}}{{\mathbf{W}}^{†}}_{\mathrm{A}}$

  (18a)

${{\Lambda }^2}_{\mathrm{A}}{{\widehat{\mathbf{U}}}^{†}}_{\mathrm{A}}={\mathbf{W}}_{\mathrm{A}}{\mathbf{m}}_{\mathrm{A}}{{\mathbf{V}}^{†}}_{\mathrm{A}}$

  (18b)

equivalent to

${\widehat{\mathbf{U}}}_{\mathrm{A}}{{\Lambda }^2}_{\mathrm{A}}{\mathbf{W}}_{\mathrm{A}}={\mathbf{V}}_{\mathrm{A}}{\mathbf{m}}_{\mathrm{A}}$

  (19a)

${{\Lambda }^2}_{\mathrm{A}}{{\widehat{\mathbf{U}}}^{†}}_{\mathrm{A}}{\mathbf{V}}_{\mathrm{A}}={\mathbf{W}}_{\mathrm{A}}{\mathbf{m}}_{\mathrm{A}}$

  (19b)

Multiplying Eq. (18b) by Eq. (19a) and Eq. (18a) by Eq. (19b) on each side, we obtain two eigenvalue equations, namely :

${{\Lambda }^2}_{\mathrm{A}}{{\widehat{\mathbf{U}}}^{†}}_{\mathrm{A}}{\widehat{\mathbf{U}}}_{\mathrm{A}}{{\Lambda }^2}_{\mathrm{A}}{\mathbf{W}}_{\mathrm{A}}={\mathbf{W}}_{\mathrm{A}}{{\mathbf{m}}^2}_{\mathrm{A}}$

  (20a)

${\widehat{\mathbf{U}}}_{\mathrm{A}}{{\Lambda }^4}_{\mathrm{A}}{{\widehat{\mathbf{U}}}^{†}}_{\mathrm{A}}{\mathbf{V}}_{\mathrm{A}}={\mathbf{V}}_{\mathrm{A}}{{\mathbf{m}}^2}_{\mathrm{A}}$

  (20b)

the resolution of which gives W_A and V_A , that is to say the hybridization matrix U_A = V_A W^†_A for each atom A we were looking for. However, it is necessary to make sure that the elements of the m_A matrix as calculated from Eq. (19a) have non-negative values, a condition which may be always ensured by changing signs in the columns of W_A initially computed from Eq. (19b) [32].

In Fig.1, we give the flow chart of the computer program to be implemented in order to determine the best possible hybridization matrix U for a molecule satisfying the criterion of maximum overlap according to Del Re. The successive steps of the calculation for a given atom, say A , are :

1. Construct the overlap matrices S_AB, S_AC …

2. Compute S_AB S^†_AB and S^†_ABS_AB, S_AC S^†_AC and S^†_AC S_AC etc …

3. Evaluate the eigenvalues λ² of the couples of AB , AC etc … product matrices

4. Select the highest eigenvalues (λ²_AB)max, (λ²_AC)max,… and the corresponding eigenvectors and form the Û_A blocks, the columns of which are the eigenvectors associated with (λ²_AB)max, (λ²_AC)max etc …

5. Form the diagonal matrix Λ from the λ squared precedent values

6. Calculate and diagonalize the Λ²a Û^†_A Û_A Λ²_A product , the eigenvectors of which are the columns of the new matrix W_A and the eigenvalues are the elements m_Ai^2.

7. Calculate and diagonalize the Û_A Λ⁴_A Û_A product, the eigenvectors of which are the columns of the new matrix V_A.

8. Form the product V_A W^†_A = U_A giving the hybridization matrix of the orbital centered on atom A (and the angles between resulting hybrids from their s and p percentages)

9. Repeat for the next atom B and so on …

The algorithm of Fig. 1 works satisfactorily as soon as atom A is able to use all its valence orbitals to form as many bond hybrids with its partners B, because a well-defined non-zero λ_Ai² value is effectively found for each hybrid. In the case of an insufficient number of partners B as in Fig.1, the zero input-values assigned to orthogonal orbitals corresponding to absent links have to be treated in different ways according to the structure of the molecule considered : i) A belongs to a conjugated system ; λ²_Ai= 0 corresponds to a (2)pπ pure atomic orbital ii) A is a heteroatom bearing a σ lone-pair, as nitrogen in amines or oxygen in carbonyls; λ²Ai = 0 has to be substituted by an appropriate non-zero value in order to have a set of hybrids correctly directed [55], as will be described in Section 2.2.2 iii) A is a radicalic center, as in trivalent carbon compound ; λ²_Ai = 0 is retained or substituted by another value, according as the species in question is a π or a σ free radical.

In passing, we will note that the algorithm first developed for hybridization can be adapted to the problem of the localization of the normal vibration modes given by the GF Wilson method [56].

For a long time, and still nowadays, hybridization has been used as a starting point for, so to say, a “linnean classification” of molecular observables ; it enables us to rationalize a large body of experimental data : Internuclear distances, bond angles, vibration frequencies and even chemical reactivity [64,65], or their theoretical counterparts coming from quantum-mechanical calculations in terms of standard s, p, d … atomic contributions. We are not willing to go back to this matter in its entirety, because it is presented in textbooks for most of these properties ; as little less standard topics, we have preferred to turn us to those properties which are known by the generic name of spin coupling constants in magnetic resonance spectroscopy.

The quantum-mechanical picture of hyperfine structures presented by the spin-spin nuclear magnetic resonance (NMR) and electron-spin resonance (ESR) spectra involves a variety of spin Hamiltonian parameters of molecular origin whose magnitude determines that of the coupling constants. In such an analysis, the most characteristic term arises from the Fermi -or contact - operator:

${\mathbf{H}}_{\mathrm{Fermi}}\propto {\displaystyle {\sum }_{\mathrm{elec}}{\displaystyle {\sum }_{\mathrm{nuc}}\mathrm{\delta }\left({\mathrm{r}}_{\mathrm{elec}}-{\mathrm{r}}_{\mathrm{nuc}}\right){\mathbf{S}}_{\mathrm{elec}}{\mathbf{I}}_{\mathrm{nuc}}}}$

(see e.g. [66]), the importance of which is connected with the values of electron densities (in NMR) or spin densities (in ESR) on the nuclei experimentally tested. The specific form of Fermi operators implies that the magnitude of the contact terms is determined by the s local character of the molecular wave function on some nuclei, i.e., by the relative importance of its s atomic components in these points. Let us add that other spin operators involved in magnetic resonance spectroscopy, such as the electron-spin and spin-spin dipole operators give, for their part, contributions determined by the p character of the wave function. In this matter, hybridization is of paramount importance for the understanding of spin coupling constants in terms of electronic structure.

The ^1JXY coupling constants between two chemically bonded nuclei X and Y in NMR give a striking example of the explanatory power of the hybridization concept, especially for the ¹³C -H and ¹³C - ¹³C couplings, where the Fermi contact term is known to be dominant over all the other types of magnetic interactions. Their experimental values can be rationalized by formulas of the form:

${}^1{\mathrm{J}}_{\mathrm{XY}}=\mathrm{k}\mathrm{sX}\mathrm{SY}+\ell \mathrm{with}\mathrm{sH}=1$

k and ℓ being parameters determined from spectroscopic data by least-square fittings in appropriate series of chemical compounds, and s the s-percentage of the atomic hybrids forming the X - Y bond. Actually, this relationship works well for the couplings across the CH and CC bonds of acetylene, ethylene and ethane, where the s fractional character of the sp, sp² and sp³ standard hybrids varies from 0.50 to 0.33 and 0.25. Of course, explicite hybridization calculations are needed for hydrocarbons having particular structures (for instance three- or four-membered cycles [67)]). The k proportionality factor is much greater than the ℓ constant, so that the latter may be ascribed to small perturbation effects not included in the Fermi contact term.

In ESR spectroscopy, the large quantity of data gathered between 1960 and 1980 has been interpreted in terms of σ and π spin densities, according to the orbital description of the unpaired electron in an independent-particle model. Within this picture, a σ radical has a singly occupied orbital belonging to the same subset as the hybridized bond and lone-pair σ orbitals, while a π radical uses an orbital built from atomic functions perpendicular to the planar or locally planar σ framework [68,69]. So it is easy to understand by symmetry reasons why the electron-spin coupling constants of the σ free radicals are dominated by the direct contribution of the contact term, the magnitude of which depends on the s character of the singly occupied orbital, whereas the couplings of the π radicals have indirect contributions due to σ–π spin-polarisation mechanisms only [70,71].

Still, the concept of hybridization is relevant even for electron-proton couplings a_i^H because their values are practically determined by the spin density ρ_i located on the next-neighbour atom i of the proton considered, for instance the unsaturated carbon C to which H is attached. These couplings are governed by relationships of the form

${{\mathrm{a}}_{\mathrm{i}}}^{\mathrm{H}}={\mathrm{Q\rho }}_{\mathrm{i}}$

where Q is a proportionality factor possibly depending on other characteristics of carbon C_i. If the spin densities are known, it is possible to derive an empirical value Q_exp for this factor using experimental data. The variation of Q_exp inside a series of related molecules enables us, by comparison with the bond angles, to see whether the usual hypothesis of atomic hybrids directed to each other has to mitigate in some cases.

To illustrate the last point, we will consider the ESR spectra coming from an unpaired electron in π electron systems of unsaturated monocycles C_nH_n. Using 1/n as spin densities ρ_i^π for carbon C_i , the ratio Q_exp = a^H/ ρ_i^π computed from the experimental data does not change appreciably, in spite of apex angles CCC deviating very much from the 120° standard value for n = 6. This fact is easily understood in the frame of the hybridization theory by assuming that Q_exp is not determined by the values of the cyclic bond angles, but by the less sensitive values predicted by the Del Re hybridization theory [72] ; in other words, the straight bond hypothesis has to be relaxed for strongly strained systems.

Let add that similar considerations cannot be developed without caution in the case of long-range spin parameters of NMR and ESR spectroscopies, because these couplings result from a delicate balance of many effects (see [73]). Their study (including possible vibrational contributions) requires much sophistification in the application of quantum mechanical methods to the evaluation of spin-hamiltonian parameters by perturbation theories at the semiempirical level (see e.g. [74]) as well as at the non-empirical one. A striking example in ESR spectroscopy is the calculation of the electron-proton spin coupling constants for vinyl, the prototype of σ free radicals [75].

3.3 Quantum-chemical flashback

Beside its various applications to structural purposes in Chemistry, the concept of hybridization has a long story in Theoretical Chemistry as an intermediate tool in the construction of realistic molecular wave functions. In the old days of Quantum Mechanics, it was confidently used to determine bond and lone-pair functions for molecular orbital and valence-bond treatments, especially in the frame of the perfect pairing approximation (see e.g. [5]). Later, this approach was revived in order to start with convenient building blocks for writing a total wave function where electronic interaction can be introduced either in a perturbative way, as in the PCILO method [76], or in a variational way passing through quasi-localized orbitals at various approximation levels of the VB and MO theories, (for a recent example see [77]). Presently, SCF-CI treatments using delocalized molecular orbitals seem to be still preferred, but it may be interesting for many reasons including Chemistry to have the possibility of replacing a set of pure s,p,d etc… functions by its equivalent in terms of hybrids adapted to the structure of the molecule in question. This is the aim of hybridization procedures, like the Del Re method. Both points of view have been exploited in the CS-INDO-CI semi-empirical method [78,79], where the basic core parameters responsible for the agreement with experiment as concerns conformations and spectra are determined on a set of hybrid orbitals, but the CI computation of the electronic spectrum itself uses pure atomic orbitals, just for convenience’s sake.

To conclude this review, we should like emphasize the fact that no serious argument can be presented for an exclusive use of pure atomic orbitals in quantum-chemical calculations, except that of the separation of the radial and angular parts of the wave function in the Hartree-Fock picture of the atoms themselves [80]. To the defenders of the traditional s, p, d … orbitals, we wish to reply that there are four coordinate systems for which the Schrödinger equation of the hydrogenic atom can be solved [81], instead of eleven for a wave equation without any potential term (see e.g. [82,83]), and that one of them, the parabolic system, gives rise to sp hybrids directly [84]. Taking this argument stricto sensu , we could be tempted to connect the origin of hybridization to the degeneracy of the s and p levels in hydrogen-like atoms ; and so its importance should gradually decrease from carbon where the 2s and 2p levels have very close orbital energies to nitrogen and oxygen atoms, in conformity with the primitive ideas of Pauling. However, we can also state that the formation of a chemical bound creates an internal electric field suggesting by its analogy to a Stark effect that the polar-coordinates picture is not really adequate for atoms in molecules. More generally, hybridization can be considered as a way to prepare a local set of orbitals belonging to some irreducible representations of a certain point group [19], in other words to break the isotropy of the atom and to raise the organization level of matter in this way[85].