| 3 | Multiplication |
Suppose you go into a store and buy seven articles for $1.00 each. The total cost is $7.00; you count $1.00 seven times. What if the articles cost $3.00 apiece? In that case, to find the total, you must count $3.00 seven times. This type of problem leads to the next step in calculating: multiplication.
IT’S A SHORTCUT
Multiplication is a shortcut for repeated addition. At one time, children learning arithmetic memorized huge multiplication tables without knowing why they’d ever need such facts. If you memorized, say, 7 times 3 is 21 (written 7 × 3 = 21), you could do calculations more quickly than if you had to go to a table and look up every multiplication fact. But not many people could tell you the reason why 7 × 3 = 21! Figure 3-1 shows why this is true: you must add up seven “threes” to get 21.
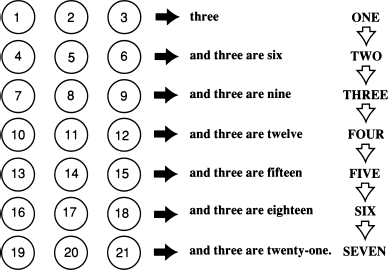
Figure 3-1
Multiplication is a form of repeated addition. In this case, seven sets of three items, all added up, gives us 21 items. We write 7 × 3 = 21.
You won’t regret it if you memorize multiplication facts up through 9 × 9 = 81, as shown in Table 3-1. These facts will be a great convenience if you know them “by heart.” Then, along with the material you will learn in the rest of this chapter, when you need to multiply two numbers together and you don’t happen to have a calculator handy, you will be able to do it without any trouble.

Table 3-1
Multiplication table from 2 × 2 up through 9 × 9. This is the sort of table you used in grade school. It’s not a bad idea to memorize it!
MAKING A TABLE
The multiplication table was one of humankind’s earliest computers: a ready way of getting answers without having to add things over and over. To understand it, you can set up a multiplication table for yourself, as shown in Table 3-1. Start with the numbers along the top and down the left side. Now, count in twos, and write the results in the next column, under “2.” Each next figure down the column is 2 more than the one above it. Now, do the same thing with the “3” column, adding 3 for each next figure down the column. Continue until you have done the “9” column.
PATTERNS IN MULTIPLICATION TABLES
If you count carefully enough, your table should turn out right. The idea behind making your own table is to convince yourself that the table is true. That way, using it will not be “cheating,” which is what some teachers used to say about students who used printed tables to solve their multiplication problems. You will be using what you have already done and verified. So, how do you verify it?
The simplest check involves looking at the odds and evens. An even number is one whose ones figure is 2, 4, 6, 8, or 0. An odd number has a ones figure of 1, 3, 5, 7, or 9. Notice that the only places in the table (called cells) where you have odd numbers are where both the number at the top of the column and the number at the left-hand end of the row are odd. If either of those numbers is even, the number in the cell is even.
In Fig. 3-2A, examine the numbers in the cells whose column or row header is equal to 5. All these numbers have a ones figure of either 0 or 5. Another thing you should notice is that any multiplication answer, called a product, can be found in two places, except where a number is multiplied by itself. For example, 3 × 7 is the same as 7 × 3, as shown at B. You see 21 in two places. This rule is true for every combination of two different numbers. It is called the commutative law for multiplication.

Figure 3-2
Some patterns in numbers make useful checks. At A, checking the fives; at B, checking by symmetry; at C, checking the nines; at D, checking by diagonals.
Another interesting thing happens in the column or row against 9, as shown at C. Notice that each successive number has one more in the tens and one less in the ones, and that adding the two digits in the product together always makes 9.
Now take the diagonal where numbers are multiplied by themselves. These numbers are called squares, and they are shown at D. You can complete the table by adding the digit 1 at the extreme upper left. Put a circle around it to indicate that 1 × 1 = 1, so you remember that the number 1 is its own square. Notice the difference between successive places down the squares diagonal:
4 − 1 = 3
9 − 4 = 5
16 − 9 = 7
25 − 16 = 9
36 − 25 = 11
49 − 36 = 13
64 − 49 = 15
81 − 64 = 17
The differences start at 3 and then keep on increasing by 2 each time: 3, 5, 7, 9, 11, 13, 15, and 17. If you want to expand this table, you can verify that the progression continues on like this for all squares, that is, for all numbers that are equal to some smaller number multiplied by itself.
Here’s another interesting quirk in the multiplication table. Notice what happens when you move upward and to the right (“northeast”) or downward and to the left (“southwest”) by one cell from a square. Going either way from 9 gives you 8. Going either way from 16 gives you 15. Going either way from 25 gives you 24, and so on. Moving one cell “northeast” or “southwest” away from the square diagonal always causes a decrease of 1.
HOW CALCULATORS MULTIPLY
The tables we have just examined only go up to 9 × 9. Years ago, children had to learn the tables up to 12 × 12, or even up to 20 × 20. What a chore! In the decimal number system, all we really need to memorize is the multiplication facts up to 9 × 9. Multiplying by 10 merely adds a zero to the end. We can expand on other facts, too. For example, 5 × 6 = 30, so it follows that 5 × 60 must be 300 and then 50 × 60 must be 3000. Whichever number you multiply by 10, the product also gets multiplied by 10 (using an extra 0).
Calculators use this fact. Actually a calculator does not multiply. It keeps on adding. But because it goes so much faster than any human can, it seems as if it arrives at the product instantly. If you enter 293 × 135 in a calculator, for example, it follows the process shown in Fig. 3-3 to arrive at the product 39,555. The calculator performs repeated addition operations to do what we humans call multiplication.

Figure 3-3
An example of the process by which a calculator multiplies two numbers.
Sometimes, people have to multiply big numbers by hand. To do this, you must multiply every part of one number, the multiplicand (as it used to be called), by every part of the other number, the multiplier. You can multiply in either order as long as you do it systematically. Figure 3-4 shows how the product 293 × 135 can be determined manually, going by hundreds, tens, and ones. We must take things in order, from left to right in each number, working with the multiplicand (293) first in each case. Most people did not learn to multiply large numbers by using this method. Maybe they would understand multiplication better if they had. At least, they’d have a better idea of how calculators work!

Figure 3-4
Here is a way to find the product 293 × 135 manually by hundreds, tens, and ones.
CARRYING IN MULTIPLICATION
When we are performing addition, carrying is a way of leaving out an extra digit, saving it for awhile, and then adding it back in later. We can do the same thing in multiplication. Figure 3-5 shows how carrying is done when we want to multiply 3,542 × 27. This can be broken down into a sum of two products: 3,542 × 7 and 3,542 × 20.

Figure 3-5
A multiplication problem that involves finding the product in two parts.
To get the first partial product, namely, 3,542 × 7, we first do the ones. That is 7 × 2 = 14; write 4 and carry 1. The tens: 7 × 4 = 28 with 1 carried to make 29; write 9 and carry 2. The hundreds: 7 × 5 = 35 with 2 carried to make 37; write 7 and carry 3. The thousands: 7 × 3 = 21 with 3 carried to make 24. This gives us 24,794.
To get the second partial product, we multiply 3,542 × 20 in the same fashion to get 70,840. Sometimes you don’t write the zero; you just move the last digit (in this case 4) over one place to the left, so it’s in the tens column. The whole thing is written down in one “piece” or algorithm, as the professional mathematicians call it. This is how people performed multiplication before they had computers.
At one time, the “new math” consisted in multiplying from the other direction. In Fig. 3-6, the same multiplication is performed in the reverse order: first the 20 and then the 7. The answer is the same either way, provided no mistakes are made. If the multiplier (that is, the second number or the lower number) has three or more digits, we must work consistently, either from left to right or from right to left.

Figure 3-6
It doesn’t matter which part of the problem we do first. We get the same answer either way.
USING YOUR CALCULATOR TO VERIFY THIS PROCESS
When you have a digital calculator, it is easy to punch in one number, then the “times sign” (×), then the other number, and finally the equals sign ( = ). Bingo, you have the answer, all complete! But this doesn’t help you see how the calculator actually does the work. Figure 3-7 can help you see that for the product 3,542 × 27.

Figure 3-7
Here is how you can use a calculator to find the product 3,542 × 27 in two parts, as shown previously by the longhand method.
Suppose we have a calculator with a single memory, which is the simplest type. Multiplying 7 by 2 gives us 14, which we enter in memory with a button labeled something like “MS” for “memory save.” Then we multiply 7 by 40, which gives us 280. Next, we add this number to the 14 with the “M+” or “memory add” button. We can read what we already have by pressing the “MR” or “memory recall” button. Finish multiplying by 7 and then press the MR button to display the first partial product of 24,794.
After that we can go on and multiply by 20. With a single memory, we don’t see the “times 20” part separately, as we do in longhand. But the final answer is the same. If you have a calculator with more than one memory, you can store each partial product in a separate memory and then add the contents of the two memories.
PUT THOSE ZEROS TO WORK!
When you multiply by longhand, don’t forget the zeros, if your multiplier has a zero in it. When you pass zero, there is no point in writing a line of zeros, because zero times anything is zero. But don’t forget that, in this case, after multiplying by 20 (the tens figure), the next one is the thousands figure, which moves to the left two places instead of one, because the multiplier has no hundreds figure. It’s a good idea to write down all the zeros in a partial product that ends with them. This can help you keep the digits in their proper places, as shown in Fig. 3-8.

Figure 3-8
In multiplication, zeros can be used to keep figures in their proper places.
When we multiply two numbers by the longhand method, we tend to use the shorter number as the multiplier because it looks like less work. Of course it really isn’t. We still have to multiply every digit in one number by every digit in the other. If you use a calculator to solve a multiplication problem straightaway by entering one number in full, then the times button, then the other number in full, and finally the equals button, you can multiply a short number by a long one or vice versa. The answer, as well as the amount of work you do, will be the same either way.
USING SUBTRACTION TO MULTIPLY
Sometimes it’s convenient to make complicated calculations in your head, instead of relying on a calculator. Although calculators don’t need shortcuts or tricks, they can be a big help when you do things in your head. Figure 3-9 shows two examples of using subtraction to help do multiplication problems. People are almost always amazed when I show them this trick, which works best when the multiplier ends in 8 or 9.

Figure 3-9
Subtraction can help us do certain multiplication problems. At A, we multiply 47,392 by 29. At B, we multiply 63,257 by 98. The examples at left show subtraction. The examples at right show the traditional addition method for comparison.
In Fig. 3-9A, we multiply 47,392 by 29. At left, the multiplier 29 is just 1 less than 30. So we multiply by 30, which is much easier than multiplying by 29. Then we subtract 1 times the original number, which is itself. Check it in the usual way as shown at right, and you will find the same answer. In Fig. 3-9B, we multiply 63,257 by 98. At left, 98 is 2 less than 100. Multiplying by 100 puts two zeros to the right of the original number. Subtract 2 times the original number in the multiplier, and you have the answer, which is verified at right.
MULTIPLYING BY FACTORS
Figure 3-10 illustrates a trick that works well with numbers that can be broken into single-digit factors. What is a factor? When two numbers are multiplied by each other to get a third number, then the first two numbers are factors of the third number. Some numbers can be split into whole-number factors, and others cannot.

Figure 3-10
An example of multiplication by factors. Here, we multiply 23,657 by 35. At left, the addition method is shown. At right, we multiply 23,657 by 5 and then multiply that product by 7, because 5 and 7 are factors of 35.
Suppose we have a multiplication problem in which the multiplier is 35. That happens to be 5 × 7. The factors of 35 are therefore 5 and 7. Instead of multiplying the whole original number by 5 and by 30 and adding the results, you can multiply first by 5 and then multiply that result by 7. As is shown here, both ways give the same answer. You can verify this on your calculator, too.
MULTIPLYING IN NONDECIMAL SYSTEMS
When you use systems that are nondecimal (not based on the number we call ten), multiplication is a little bit complicated. Some calculators are equipped to make conversions to nondecimal systems, but you need to know what to do if your calculator can’t do that and you encounter such a problem.
The “pounds and ounces” or “English” weight measurement system is nondecimal, with 16 ounces to the pound. Suppose you want to find 25 times 1 pound 3 ounces. You can multiply the pounds by 25, then multiply the ounces by 25, and then just stick them together and say that the product is 25 pounds 75 ounces. But that isn’t the way you’d normally express weight! The ounces part should always be less than 16. If it goes to 16 or more, you should add 1 to the pounds part of the figure and subtract 16 from the ounces part. You might have to do this more than once to get an ounces figure of less than 16. As you find the product 25 times 1 pound 3 ounces, doing this procedure four times converts the 75 ounces to 4 pounds and 11 ounces (Fig. 3-11). Then you add the 4 pounds to the 25 pounds, so the total is 29 pounds 11 ounces.

Figure 3-11
A multiplication problem involving weight in the “pounds-and-ounces” system. Here, we multiply 25 times 1 pound 3 ounces. Note that a pound contains 16 ounces.
Figure 3-12 shows another nondecimal multiplication problem. The ”yards-feet-inches” or “English” length measurement system defines 12 inches to the foot and 3 feet to the yard. Where necessary, we have to make conversions between inches, feet, and yards. How long must a piece of lumber be in order to get five 10-inch pieces without any waste when we cut it into equal pieces? The answer is 50 inches, but we would usually say it is 4 feet 2 inches. We could also say it is 1 yard 1 foot 2 inches.

Figure 3-12
Multiplying lengths. We need a piece of lumber 4 feet 2 inches long if we want to cut it into five 10-inch pieces without any waste.
In the same way, if you multiply a measure by a large number, it is usually convenient to change the unit of measure in which we express it, as shown by the example in Fig. 3-13. Suppose a motor crankcase takes 3 pints of oil. How much oil will you need for 250 motors of this same kind? You can multiply 3 pints by 250 and get an answer of 750 pints. But quantities this large are usually given in gallons, not in pints. Remembering that 8 pints make a gallon, you can proceed to count backward in eights. From 750, 8 can be subtracted 93 times, and when you are all done with this process, you’ll have 6 pints (or 3/4 of a gallon) left over. But that is a tedious way to do it! You must be thinking there’s an easier way, and you are right. We can use division. We’ll learn about that in Chap. 4.

Figure 3-13
Multiplying liquid measures. This requires that we subtract 8 pints over and over and over, dozens of times! In the next chapter we'll learn how division can make it easier to do problems like this.
QUESTIONS AND PROBLEMS
This is an open-book quiz. You may refer to the text in this chapter (and earlier ones, too, if you want) when figuring out the answers. Take your time! The correct answers are in the back of the book.
1. Multiply the following pairs of numbers as shown. Check your results by using the left-hand number as the multiplier in each case.
(a) 357 × 246
(b) 243 × 891
(c) 24 × 36
(d) 37× 74
(e) 193 × 764
(f) 187 × 263
2. Multiply the following pairs by using subtraction to make the work simpler. Verify your results by the more usual method.
(a) 2,573 × 19
(b) 7,693 × 28
(c) 4,497 × 18
(d) 5,396 × 59
(e) 7,109 × 89
3. Multiply the following pairs by using factors of the second number. Check your results by the more usual method.
(a) 1,762 × 45
(b) 7,456 × 32
(c) 8,384 × 21
(d) 9,123 × 63
(e) 1,024 × 28
4. An airline runs four flights per day between two cities, except on Sundays when it runs only two flights. How many flights per week is this?
5. The flight described above is also made only twice on 12 public holidays during the course of a year. How many flights are made in a period of a year based on exactly 52 weeks?
6. A mass-produced item costs 25 cents each to make and $2.00 to package. The packet cost is the same, no matter how many items are in it. Determine the cost for packets of
(a) 1
(b) 10
(c) 25
(d) 100
(e) 250
(f) 1,000
(g) 5,000
(h) 10,000
7. If you ignore the manufacturing cost in problem 6, how much is saved by packing 500 items in packets of 250 as opposed to packets of 100?
8. On a commuter train, single tickets sell for $1.75. You can buy a 10-trip ticket for $15.75. How much does this save over the single-ticket rate if you take 10 trips?
9. On the same train, a monthly ticket costs $55. If a commuter makes an average of 22 round trips a month, how much will he save by buying a monthly ticket as compared with paying for every trip individually?
10. Suppose that a corporation offered a 10-hour-a-week part-time employment contract beginning at $500 a month, with a raise of $50 a month every year for five years. The contract expired after the sixth year. Employees bargained for a starting figure of $550 a month, with a raise of $20 a month every six months. Which rate of pay was higher at the beginning of the sixth year? Which rate resulted in the greatest total earnings per employee for six years? By how much?
11. A manufacturer prices parts according to how many are bought at once. The price is quoted per 100 pieces in each case, but the customer must take the quantity stated to get a particular rate. The rates are $7.50 apiece for 100, $6.75 apiece for 500, $6.25 apiece for 1,000, $5.75 apiece for 5,000, and $5.50 apiece for quantities over 10,000. The rate for any in-between quantity is based on the next-lower number. What is the difference in total cost for quantities of 4,500 and 5,000 parts?
12. Small parts are counted by weighing. Suppose 100 of a particular part weigh 2-1/2 ounces and you need 3,000 of the parts. What is the total weight?
13. A bucket that is used to fill a tank with water holds 4 gallons. To fill the tank, 350 bucketfuls are required. What is the tank’s capacity?
14. A freight train has 182 cars each loaded to the maximum which, including the weight of the car, is 38 tons. What is the total weight that the locomotive has to haul?
15. A car runs 260 miles on one tank of gas. It has an alarm that lets the driver know when it needs refilling. If a particular journey required 27 fillings and at the end, the tank was ready for another, how long was the journey?
16. Two railroads connect the same two cities. The first railroad company charges 10 cents per mile, and the second company charges 15 cents per mile. The distance between the cities is 450 miles by the first railroad, but only 320 miles by the second. Which company offers the cheaper fare? By how much?
17. One airline offers rates based on 14 cents per mile for first-class passengers. Its distance between two cities is 2,400 miles. Another airline offers 10 cents a mile for coach, but uses a different route, marking the distance at 3,200 miles. Which fare is cheaper? By how much?
18. The first airline in the scenario of problem 17 offers a family plan. Each member of a family, after the first member, pays a rate that is based on 9 cents per mile. Which way will be cheaper for a family (a) of 2 and (b) of 3? By how much?
19. A health specialist recommends chewing every mouthful of food 50 times. One ounce of one food can be eaten in 7 mouthfuls. A helping of this food consists of 3 ounces. How many times will a person have to chew this helping to fulfill the recommendation?
20. An intricate pattern on an earthenware plate repeats nine times around the edge of the plate. Each pattern has seven flowers in the repetition. How many flowers are around the edge of the plate?
..................Content has been hidden....................
You can't read the all page of ebook, please click here login for view all page.
